Polyliner
by: Eva WohllebenPolyliner
Is there a periodic table of geometric forms?
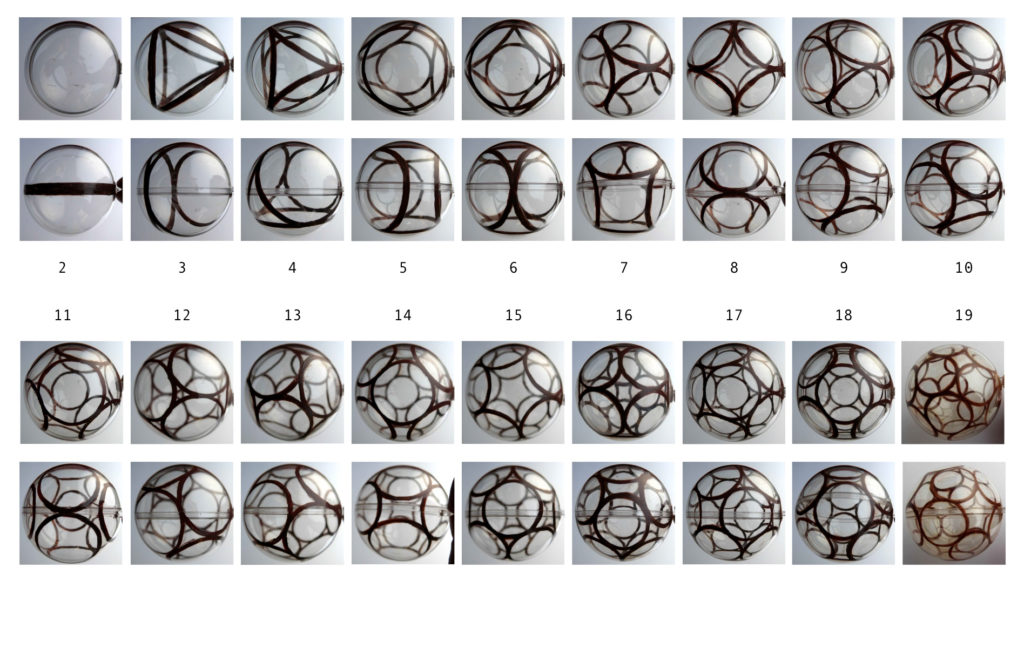
In the project „Polyliner“ a class of new geometric solids, introduced by Eva Wohlleben in 2015, is further explored and cataloged. Objects interpreted purely graphically in mathematics, are now established spatially. In the process, the principle of duality, in which points and planes exchange their roles, is applied to non-polyhedral geometric solids. Thus, dual pairs are created whose figures are reminiscent of plant structures. With their arched facettes and curved ridges they still relate to each other in the framework of polyhedral geometry. In drawing and model making, the individual polyliner forms are examined for their potential as intersection points of kinship lines, to be grouped in ordering schemes. The formulations aim at a periodic table of geometric forms based on the properties of symmetry, self-duality and chirality, as well as on triplets of transition forms and the outline of corresponding loops. This extended repertoire of mathematical structures, together with the emerging catalog of notations, offers points of contact with other scientific disciplines, for example, in the dialogue on orbital models, growth processes in botany, and ideas about fine structures of matter.
Eva Wohlleben focuses her theoretical and artistic research on mathematical issues: units of flexible spacial tesselation (Corpuscle), 3D-representatives of numbers (Polyliner) and the coherence of intersected space in its elastic processes (Inversion Kinematics). In the water research lab of Herrischried, she studies the structural relationship between form and movement by a hydro-morphological and picture-forming method. She exhibits in Art&Science projects, publishes and lectures at international conferences and gives seminars on projective and experimental geometry.
Polyliner
Gibt es ein Periodensystem geometrischer Formen?
Im Projekt „Polyliner“ wird eine von Eva Wohlleben 2015 eingeführte Klasse neuer geometrischer Körper weiter erforscht und katalogisiert. In der Mathematik rein grafisch interpretierte Objekte werden räumlich ausgeführt. Dabei wird das Prinzip der Dualität, in dem Ecken und Flächen ihre Rollen tauschen, auf nicht-kristallgeometrische Formen angewandt. So entstehen Dualpaare, deren Körper an pflanzliche Strukturen erinnern, und die mit ihren gewölbten Flächen und gekrümmten Graten weiterhin nach den Regeln der Kristallgeometrie in Beziehung zueinander treten. In Zeichnung und Modellbau werden die einzelnen konkreten Polylinerformen auf ihr Potential als Kreuzungspunkte von Verwandtschaftslinien untersucht, um in Ordnungsschemata gruppiert zu werden. Die Entwürfe zielen auf ein Periodensystem geometrischer Formen ab, das auf den Eigenschaften von Symmetrie, Selbstdualität und Chiralität, sowie auf Tripletts von Übergangsformen und der Gliederung korrespondierender Schleifen-Linien basiert. Dieses erweiterte Repertoire mathematischer Strukturen bietet mit dem entstehenden Notationskatalog Anknüpfungspunkte zu einer Interaktion mit anderen wissenschaftlichen Disziplinen, zum Beispiel über Orbitalmodelle, Wachstumsprozesse in der Botanik und Vorstellungen zu Feinstrukturen von Materie.
Eva Wohlleben fokussiert ihre theoretische und künstlerische Forschung auf mathematische Fragestellungen: Einheiten flexibler räumlicher Parkettierung (Korpuskelgeometrie), 3D-Repräsentanten von Zahlen (Polyliner) und die Kohärenz des geteilten Raums in seinen elastischen Prozessen (Inversionskinematik). Im Wasserforschungslabor Herrischried untersucht sie mit einer hydro-morphologischen, bildschaffenden Methode die strukturelle Beziehung zwischen Form und Bewegung. Sie stellt in Art&Science Projekten aus, publiziert und referiert auf internationalen Konferenzen und gibt Seminare zur projektiven und experimentellen Geometrie.